.

Griechische Mathematik: Heron von Alexandria

Heron of Alexandria contributed to mathematics but he had not the mathematical “quality” of Euclid.

Euclid’s definitions of the elementary geometric entities—point, straight line, plane—at the beginning of the Elements have long presented a problem. Their nature is in sharp contrast with the approach taken in the rest of the book, and continued by mathematicians ever since, of refraining from defining the fundamental entities explicitly but limiting themselves to postulating the properties which they enjoy. Why should Euclid be so hopelessly obscure right at the beginning and so smooth just after? The answer is: the definitions are not Euclid’s. Toward the beginning of the second century A.D. Heron of Alexandria found it convenient to introduce definitions of the elementary objects (a sign of decadence!) in his commentary on Euclid’s Elements, which had been written at least 400 years before. All manuscripts of the Elements copied ever since included Heron’s definitions without mention, whence their attribution to Euclid himself. The philological evidence leading to this conclusion is quite convincing.
Sandro Graffi Review of “ La Rivoluzione Dimenticata” (The Forgotten Revolution) Lucio Russo Feltrinelli, Milan, 1996

Heron’s Formula
Let a,b,c be the sides of a triangle, and let A be the area of the triangle. Heron's formula states that A*A = s(s-a)(s-b)(s-c), where s = (a+b+c)/2. The actual origin of this formula is somewhat obscure historically, and it may well have been known for centuries prior to Heron. For example, some people think it was known to Archimedes. However, the first definite reference we have to this formula is Heron's. His proof of this result is extremely circuitious, and it seems clear that it must have been found by an entirely different thought process, and then "dressed up" in the usual synthetic form that the classical Greeks preferred for their presentations.

Prof wins award for 2000-year-old formula Is a 2000-Year-Old Formula Still Keeping Some Secrets? The American Mathematical Monthly, 107, No. 5, May 2000, 402-415.

(We introduce the reader to heretofore untold secrets of Heron's ancient formula giving the area of a triangle in terms of its three sides. You will discover that it works even for "impossible" triangles, where one side is longer than the sum of the other two. In fact, the formula turns out to be an excellent tour guide, leading us to triangles in space-time (as in relativity) and beyond, into "anti-Euclidean" space. You will learn that one normally sees less than 10% of all triangles, like the tip of an iceberg. We will "lift the iceberg out of the water", exposing all those triangles. For any of them, we can know the full suite of vital statistics--side lengths, angles, area, . .http://www.maa.org/pubs/monthly_may00_toc.html )

For a proof see Dr. McCrory Foundation of Geometry lecture:

An algebraic proof of Heron's Formula
A geometric proof of Heron's Formula. This proof is based on Heron's proof of the formula in Metrica.
A trigonometric proof of Heron's Formula

A triangle with integer edge lengths and integer area is called Heronian. Example a Heronian triangle: a = 3, b = 4, c = 5 has an area A = 6.

Heron's Formula can be written as an equation for the Area using x = A as :

x² + a⁴+ b⁴ + c⁴ – 2a²b²– 2a²c²– 2b²c² = 0

Can the equation be extended to other polygons in two dimensions? The answer is NO! The idea is that any polygon can be triangulated but only the original edges of the polygon finally have to remain. Very strange 2000 years after Heron's formula mathematicians were able to find a generalization of Heron's equation for polyhedra. In 1996 Idzhad Kh. Sabitov from the Moskau University together with Anke Walz and Robert Connelly showed that it is possible to find a corresponding formula for a polyhedron, in which the unknown x is the volume and the parameters are the sides of the polyhedron. In three dimensions a polyhedron can be split into tetrahedra and like a miracle the equations can be simplified so that only the edges of the original polyhedron remain. The formula although is very complex, for example of 16^th degree for a octahedron but an algorithm exists for other polyhedra to find the corresponding equation.

Folding & Unfolding: Unfolding Polyhedra (2.4 MB Powerpoint)

Idzhad Kh. Sabitov - Solution of polyhedra

Heron's division of a triangle

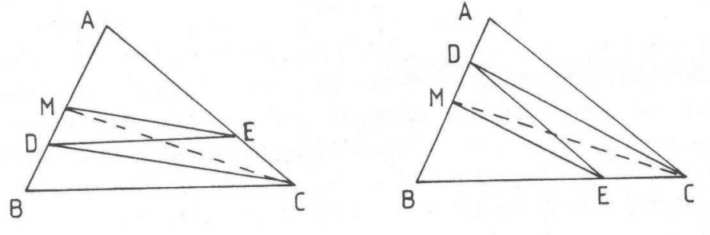
Heron provided a solution in Metrica for the geometric problem to divide a triangle in two pieces of the same area given a point D on a side of the triangle. If this point is on the middle of the side (point M) then the line MC divides the triangle in two pieces of the same area. For some other point D first get the parallel ME of the line DC. This parallel cuts the triangle in the point E. Then ED is the line that cuts the triangle in two pieces with the same area.

Heron’s square root iteration Formula

Heron described a method to calculate the square root of a number using some initial approximation. If repeated the iteration converges to the square root of the number. This method was probably also known to the Babylonians.

Heron's iterative method to calculate the square root of a “positive” number a sqrt(a).

x_n+1= ½(x_n + a /x_n )

a : number for which we want to find its square root
x_n : n-th iteration value of the square root of a
Initial value x₀

if x_n approximates sqrt(a) to d decimal places, then x_n+1 approximates qrt(a) to 2d decimals, ie each iteration doubles the number of digits of accuracy. The fact that the error in approximation is approximately the square of the error in the previous step is why the convergence of Heron's algorithm for the calculation of the square root is said to be quadratic.

Example: calculation of the square root of a = 23

Initial value: x₀ = 6
x₁: 4.9167 x²₁: 24.1739
x₂: 4.7973 x²₂: 23.0141
x₃: 4.7958 x²₃: 22.9997

...etc

Initial value: x₀ = 386

x₁: 193.0298
x₂: 96.5745
x₃: 48.4063
x₄: 24.4407
x₅: 12.6909
x₆: 7.2516
x₇: 5.2117
x₈: 4.8124
x₉: 4.7959
x₁₀: 4.7958

Result: The further the initial value x₀ is from the square root the more iterations are required.

One can consider that Herons method is just Newtons method of solving the solution f(x) = a -x²

since we get x – f(x) / f ' (x) = ½( x + a/x )

Heron’s cubic root iteration Formula

In Book III of Metrica Heron gives the cube root of 100.

Deslauriers and S Dubuc say that Heron used a iteration formula that provides approximation with an lower and upper bound a, b of the cube root of a number N:

a + b d/(b d + aD)(b - a),

where

a³ < N < b³,

d = N - a³,

D = b³ - N.

Heron’s criterion

http://www.math.ubc.ca/~hoek/Teaching/Heron/Herocrit.html

LINKS

Ancient Square Roots

G. Deslauriers and S. Dubuc, Le calcul de la racine cubique selon Héron, Elem. Math. 51, 28-34, 1996.

Thomas L. Heath, A History of Greek Mathematics, 2 vol. (1921, reprinted 1993).

Heron's Formula and Brahmagupta's Generalization